Логарифмические неравенства и их системы

    Неравенства вида log_ax > b (log_ax ≥ b) или log_ax < b (log_ax ≤ b), где a > 0, a ≠ 1, называются простейшими логарифмическими неравенствами.

    Решение логарифмических неравенств основано на строгой монотонности логарифмической функции. Известно, что при основании, большем единицы, логарифмическая функция возрастает; при положительном основании, меньшем единицы, логарифмическая функция убывает.

Неравенство вида

log_af(x) > b

эквивалентно следующим системам неравенств:

1. при a > 1   f(x) > 0, f(x) > a^b;
2. при 0 < a < 1  f(x) > 0, f(x) < a^b.

Неравенство вида

log_af(x) < b

эквивалентно следующим системам неравенств

1. при a > 1   f(x) > 0, f(x) < a^b;
2. при 0 < a < 1  f(x) > 0, f(x) > a^b.

Пример. 

Решить неравенство log₈(x² - 4x + 3) < 1.

Решение. 

Так как основание логарифма больше единицы (а = 8), то данное неравенство эквивалентно системе:

 или  

Каждое неравенство решим методом интервалов.

х² - 4x + 3 = 0 при х₁ = 1, х₂ = 3. Определяя знаки, получим:

х² - 4x - 5 = 0 при х₁ = -1, х₂ = 5. Определяя знаки, получим

Совмещая промежутки, имеем:

Таким образом, x(-1; 1)(3; 5).

Ответ: x(-1; 1)(3; 5).

Неравенство вида

log_af(x) > log_ag(x)

эквивалентно следующим системам неравенств:

• при a > 1   f(x) > 0, g(x) > 0, f(x) > g(x);
• при 0 < a < 1  f(x) > 0, g(x) > 0,   f(x) < g(x).

Неравенство вида

log_af(x) < log_ag(x)

эквивалентно следующим системам неравенств

• при a > 1   f(x) > 0, g(x) > 0, f(x) < g(x);
• при 0 < a < 1  f(x) > 0, g(x) > 0, f(x) > g(x).

Пример. 

Решить неравенство: log_0,2(x² + 6x + 8) > log_0,2(5x + 10).

Решение. 

Основание логарифмической функции меньше 1 (a = 0,2). Поэтому, выписывая области определения выражений левой и правой частей неравенства и пользуясь свойством монотонности, получим равносильную систему:

Решение неравенств второй степени методом интервалов:

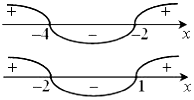
Совмещая промежутки, получим:

Ответ: (-2;1).

    Более сложные логарифмические неравенства сводятся к простейшим методами, аналогичными используемым при решении логарифмических уравнений.

Пример. 

Решить неравенство: 

Решение. 

Переходя к основанию 2 в выражении, стоящем в правой части данного неравенства, получим:

  

Теперь перейдем к равносильной системе:

Решение встречающихся квадратичных неравенств провели методом интервалов:

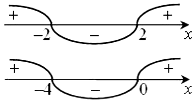
Совмещая промежутки, получим 0 < x < 2.

Ответ: (0; 2).

Пример. 

Решить неравенство x^lgx > 10.

Решение. 

Так как выражения, стоящие в левой и правой частях неравенства положительны, то для решения прологарифмируем обе части по основанию 10. Получим равносильное исходному неравенство: 

lgx^lgx > lg10 или, пользуясь свойствами логарифмов lg²x - 1 > 0.

Обозначая t = lgx, решим неравенство t² - 1 > 0:

то есть t < -1 или t > 1.

Решая неравенства lgx < -1, а также lgx > 1, имеем соответственно:

.

.

Ответ: (0; 0,4)(10;+).

    Если в неравенстве встречается логарифмическая функция, содержащая неизвестное в основании, то, как правило, следует рассматривать два случая:

• когда основание больше 1
• когда основание положительно, но меньше 1.

Неравенство с переменным основанием можно также решать, используя формулы перехода к новому, не содержащему неизвестное, основанию.

Пример. 

Решить неравенство log_x-3(x² - 4x + 3) < 0.

Решение. 

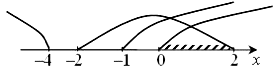
Так как основание логарифма содержит переменную, то рассмотрим два случая x - 3 > 1 и 0 < x - 3 < 1.

Если основание логарифма больше одного, то пользуясь свойством монотонности с учетом О.Д.З., получим:

Решая неравенства методом интервалов, получим:

Совмещаем промежутки и убеждаемся, что данная система не имеет решений.

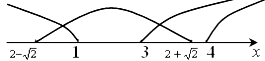
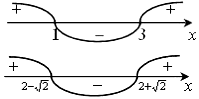
Рассмотрим второй случай, если 0 < x - 3 < 1. В этом случае получаем систему:

Совмещая промежутки, получаем:

Ответ: (2 + ; 4).