Дифференцирование и интегрирование показательных и логарифмических функций

Число е. Функция у = е^х, ее свойства, график, дифференцирование

      Рассмотрим показательную функцию у = а^х, где а > 1. Для различных оснований а получаем различные графики (рис. 1-3), но можно заметить, что все они проходят через точку (0; 1), все они имеют горизонтальную асимптоту у =0 при x→, все они обращены выпуклостью вниз и, наконец, все они имеют касательные во всех своих точках.

      Проведем для примера касательную к графику функции у = 2^x в точке х = 0 (рис1) Если сделать точные построения и измерения, то можно убедиться в том, что эта касательная образует с осью х угол 35° (примерно).

y = 2^x

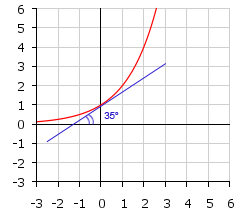
рис 1

y = 3^x 

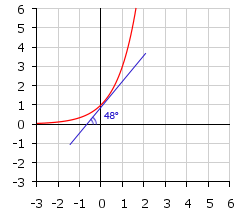
рис 2

y = 10^x

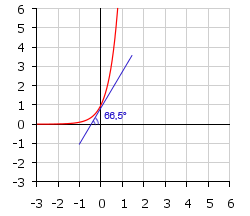
 рис 3

      Теперь проведем касательную к графику функции у = 3^x тоже в точке х = 0 (рис. 2). Здесь угол между касательной и осью х будет больше - 48°. А для показательной функции у = 10^x в аналогичной ситуации получаем угол 66,5° (рис. 3).

      Итак, если основание а показательной функции у = а^х постепенно увеличивается от 2 до 10, то угол между касательной к графику функции в точке х = 0 и осью абсцисс постепенно увеличивается от 35° до 66,5°. Логично считать, что существует основание а, для которого соответствующий угол равен 45°. Это основание должно быть заключено между числами 2 и 3, поскольку для функции у = 2^х интересующий нас угол равен 35°, что меньше, чем 45°, а для функции у = 3^x он равен 48°, что уже немного больше, чем 45°. Интересующее нас основание принято обозначать буквой е. Установлено, что число е - иррациональное, т.е. представляет собой бесконечную десятичную непериодическую дробь: e = 2,7182818284590...; на практике обычно полагают, что e = 2,7.

 y = e^x

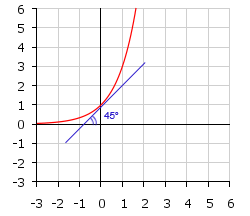
рис 4

     График функции у = е^х изображен на рис. 4. Это - экспонента, отличающаяся от других экспонент (графиков показательных функций с другими основаниями) тем, что угол между касательной к графику в точке х = 0 и осью абсцисс равен 45°.

Свойства функции у = е^х : 

1. D(f) = (-; +);
2. не является ни четной, ни нечетной;
3. возрастает;
4. не ограничена сверху, ограничена снизу;
5. не имеет ни наибольшего, ни наименьшего значений;
6. непрерывна; 
7. E(f) = (0; +);
8. выпукла вниз;
9. дифференцируема.

Производная логарифмической функции

      Начнем с производной логарифмической функции y = loga x, где основание a больше нуля и не равно единице: a > 0,  a ≠ 1. Согласно определению производной, дадим аргументу x приращение Δx > 0, причем предположим, что x + Δx > 0. Логарифмическая функция получит соответствующее приращение y, равное  Δy = log_a(x + Δx) - log_ax.

      Разделим обе части равенства на Δx - 

Обозначим  Тогда последнее соотношение можно переписать в виде

Используя свойство логарифма степенной функции, получаем: 

     Полагая Δx → 0 (в этом случае h → ), находим предел отношения приращений, т.е. производную логарифмической функции:

     Здесь мы использовали свойство предела от сложной функции, учитывая, что логарифмическая функция является непрерывной. Предел в квадратных скобках равен знаменитому числу e, которое приблизительно составляет 2.718281828... (2.7, затем два раза год рождения Л.Н.Толстого):

= e ≈ 2,718281828459...

Следовательно, производная логарифмической функции имеет вид

По формуле перехода к новому основанию логарифма, имеем:

Таким образом,

y'(x)=(log_ax)' = .

В случае a = e мы получаем натуральный логарифм, производная которого выражается формулой (ln x)' = 1/x. 

 

Отметим еще один важный частный случай − производную десятичного логарифма:

где число M равно M = lg e = 0,43429... 

Производная показательной функции

Поскольку показательная функция с основанием a (a > 0, a ≠ 1) и логарифмическая функция с тем же основанием образуют пару взаимно-обратных функций, то производную показательной функции можно найти с помощью теоремы о производной обратной функции.

 

Пусть дана пара взаимно-обратных функций y = f (x) = a x и x = φ(y) = loga y. Тогда

В частном случае a = e производная равна самой функции:

.

В примерах, приведенных ниже, найти производную заданной функции. 

Пример 1.

Решение.

Дифференцируем как частное двух функций:

 где x > 0. 

Пример 2.

Решение.

Дифференцируя данную показательную функцию как сложную, находим

Пример 3.

y = x ln x − x.

Решение.

Используя правила дифференцирования произведения функций и разности функций, получаем:

y'(x) = [xlnx - x]' = (xlnx)' - (x)' = (x)'lnx + x(lnx)' - (x)' = 1*lnx + x *  - 1 = lnx + 1 - 1 = lnx   (x > 0).

Пример 4.

 y = log2 cos x.

Решение.

Дифференцируя как сложную функцию, имеем

Данная функция определена при условии cosx > 0,   + 2n < x <  + 2n, nZ.

Пример 5.

Решение.

Пример 6.

Решение.

Применяя линейные свойства производной и правило дифференцирования сложной функции, получаем

В данном примере функция определена при x > 0. 

Пример 7.

y = log₃ (4x²).

Решение.

Пример 8.

Решение.

По правилу дифференцирования сложной функции находим

y'(x)=lntg=

Упрощаем:

Применив формулу двойного угла , получаем окончательный ответ

Пример 9.

y(x) = (ln³x + 3ln²x + 6lnx + 6)

Решение.

Сначала возьмем производную от произведения функций:

Дифференцируя отдельные члены и упрощая, получаем:

 Пример 10.

Решение.

Дифференцируя как сложную функцию и упрощая, получаем следующее выражение для производной:

Отметим, что заданная функция существует при x > 0. 

Вопросы к конспектам